Search: keyword:left-narrow
|
| |
|
| BP559 |
| Cross section of a cube vs. not cross section of a cube |
|
| ?
 |
|
|
| |
| |
|
|
| |
|
| BP569 |
| Triangular number of dots vs. non-triangular number of dots |
|
| |
|
|
|
|
COMMENTS
|
All examples in this Problem are groups of black dots.
The nth triangular number is the sum over the natural numbers from 1 to n, where n > 0. Note: 0 is the 0th triangular number. The first few triangular numbers are 0, 1, 3 (= 1+2) and 6 (= 1+2+3) |
|
|
CROSSREFS
|
Adjacent-numbered pages:
BP564 BP565 BP566 BP567 BP568 * BP570 BP571 BP572 BP573 BP574
|
|
|
KEYWORD
|
nice, precise, allsorted, notso, number, math, left-narrow, left-null, help, preciseworld
|
|
|
WORLD
|
dots [smaller | same | bigger]
|
|
|
AUTHOR
|
Leo Crabbe
|
| |
|
|
| |
|
| BP850 |
| Shape can be maneuvered around the corner vs. not so. |
|
| |
|
| |
| |
|
|
| |
|
| BP856 |
| Compound shape would hit the dot if rotated vs. not so. |
|
| |
|
| |
| |
|
|
| |
|
| BP902 |
| This Bongard Problem vs. anything else. |
|
| |
|
|
|
|
COMMENTS
|
Although this Bongard Problem is self-referential, it's only because of the specific phrasing of the solution. "BP902 vs. anything else" would also work. The number 902 could have been chosen coincidentally. |
|
|
CROSSREFS
|
See BP953, BP959.
Adjacent-numbered pages:
BP897 BP898 BP899 BP900 BP901 * BP903 BP904 BP905 BP906 BP907
|
|
|
KEYWORD
|
notso, meta (see left/right), links, left-self, left-narrow, left-finite, left-full, right-null, right-it, invalid, experimental, funny
|
|
|
CONCEPT
|
self-reference (info | search),
specificity (info | search)
|
|
|
WORLD
|
everything [smaller | same]
zoom in left (bp902)
|
|
|
AUTHOR
|
Leo Crabbe
|
| |
|
|
| |
|
| BP922 |
| One row is rearranged to make the other by swapping an odd number of object pairs vs. one row is rearranged to make the other by swapping an even number of object pairs. |
|
| |
|
| |
| |
|
|
| |
|
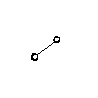
| BP932 |
| Every vertex is connected to every other vs. vertices are connected in a cycle (no other connections). |
|
| ?
 | ?
 |
|
|
|
|
|
COMMENTS
|
Complete graphs with zero, one, two, or three vertices would be ambiguously categorized (fit in overlap of both sides).
Left examples are called "fully connected graphs." Right examples are called "cycle graphs." |
|
|
CROSSREFS
|
Adjacent-numbered pages:
BP927 BP928 BP929 BP930 BP931 * BP933 BP934 BP935 BP936 BP937
|
|
|
KEYWORD
|
precise, left-narrow, right-narrow, both, preciseworld
|
|
|
CONCEPT
|
graph (info | search),
distinguishing_crossing_curves (info | search),
all (info | search),
loop (info | search)
|
|
|
WORLD
|
connected_graph [smaller | same | bigger]
|
|
|
AUTHOR
|
Aaron David Fairbanks
|
| |
|
|
| |
|
| BP935 |
| Shapes have equal area vs. not so. |
|
| |
|
| |
| |
| |
|
|
|
|
|
|
|
|